5D Mk IV – EF 70-200mm L II – 2x Doubler – 1/400, f/5.6, ISO 100

I was looking up at the sky a few days ago and saw some contrails which got me thinking this would be a good Photo of the Day. It’s also a good time to brush up on some Euclidian Geometry! Why you ask? Well, as I looked at the contrails in the sky, I realized those planes were miles away. I could barely see them with the naked eye, but with a 400mm focal length, I should be able to see them much better. Then I realized I could calculate a pretty good estimate as to how far away it was from me.

I needed a pretty nice sky with minimal clouds and had my opportunity this morning. The plane in the pic appears to be a commercial airliner. The sky was bright blue with no clouds. The plane was traveling west and left a beautiful contrail. I set the focal length to 400mm and snapped the pic.

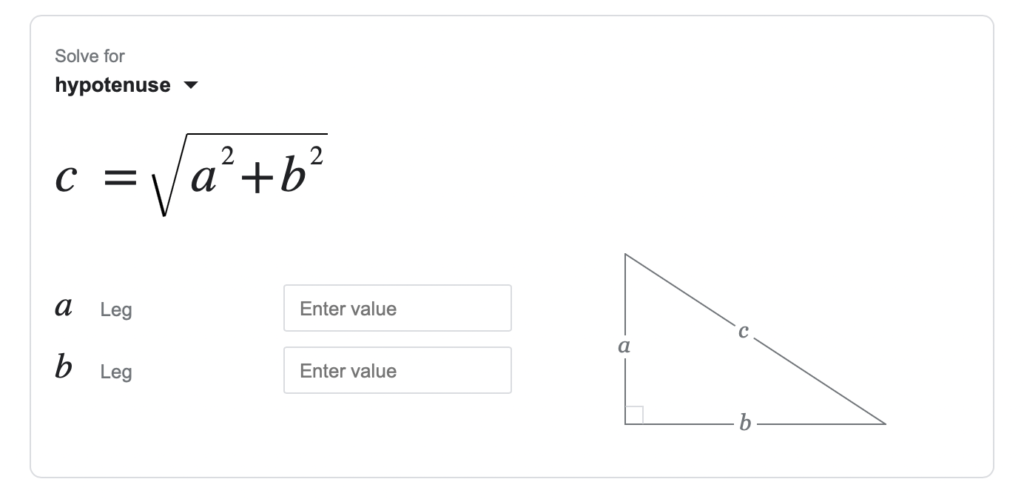
Now for the geometry. If you remember, using the Pythagorean Theorem, we can calculate the hypotenuse (side ‘c’ in the pic below) of a triangle. Side ‘a’ in the pic below represents the altitude of the plane. Commercial jets flying west, fly at even flight levels. We can make an assumption that it is flying at 36,000 ft (FL360). The harder part was estimating side ‘b’ – the ground distance of the plane from me. For simplicity sake, it was obvious the plane was well on the other side of Brookston so we’ll say 5 miles (26,400 ft). We can plug our numbers into this website. This calculates side ‘c’ to be 44,642 ft or 8.45 miles away from me.